Electrician Math: Life-Saving Skills

Electrician math is vital to your career, maybe even your life. When you construct a building that will last, you have to start with a strong foundation. No one sees the foundation when the building is finished, but it is an essential part of the structure. Electrical formulas and concepts are the foundation you need to be a successful electrician. 

Many people don’t even realize how important math is in their lives. You use it in just about everything you do. From measuring the coffee grounds and water for your coffee in the morning to counting your money at the grocery store – everything involves math in some shape or form. 

This is especially true for an electrician. You are constantly calculating measurements and voltages. You will always be using basic arithmetic (mostly fractions) to calculate many of these measurements. 

You will need to know basic right angle trigonometry when bending conduit, in order to find the correct angle and the necessary distance between bending points. Just about every job you do will involve some type of math. 

As you study electrical circuits, you will find that a solid foundation in math will help you understand the NEC and its provisions. 

The electrician math unit you will study, as part of the classroom training you need to complete your electrician’s apprenticeship, includes electrical fundamentals and an explanation of how electrical meters work in order to help you visualize how they are used in practice. 

As you continue with your electrical studies, you will be amazed at how often the basics you learned in your math unit will return. Ohm’s law and the related electrical formulas are the foundation of all electrical circuits. 

Order of Operations

Does the acronym BEDMAS (or PEDMAS) give you high-school flashbacks? This term, which breaks out into brackets (or parenthesis), exponents, division, multiplication, addition, subtraction, tells us in which order to solve an equation. For example: 

(1+1)*2 is different from 1+1*2 

(1+1)*2 = (2)*2 = 4 

1+1*2 = 1+2 = 3 

Keep this in mind, especially when typing numbers into your calculator. A missed bracket can totally change your result. 

Changing a Fraction to a Decimal

Students grasp ideas and concepts at different rates. You may find parts of your math unit as a simple review of what you already know, or you may find you need a great deal of concentration to fully understand some things. 

Either way, make certain you do fully understand each concept before moving on to the next. This way you will build a solid foundation that will help you throughout your career. 

To change a fraction into a decimal, divide the top number (numerator) by the bottom number (denominator). 

For example: 

1/6 = 0.166 

Changing a decimal to a fraction can be trickier, and really only works with whole numbers. Here are some of the most common fractions and their decimals: 

1/2 = 0.5 

1/4 = 0.25 

1/3 = 0.333 

3/4 = 0.75 

2/3 = 0.666 

Percentages

Values are often displayed as percentages. One hundred percent is equal to the entire value, 50 percent is half, and 25 percent is a quarter of the total value. Multiplying or dividing a percentage is easier when you first convert the value into a decimal and use the new number in your calculation. 

To change a percentage to a decimal, drop the % and move the decimal 2 places to the left. 

For example: 

45.2% = 0.452 

To figure out a percentage, divide the partial amount by the whole amount, then multiply by 100. 

For example: 

A 15A breaker is loaded with a 12A load. To what percentage is the breaker loaded? 

12A/15A x 100 = 80% 

Percentages can be higher than 100. 

For example: 

A wire is rated for 50A but is carrying a measured load of 130A. What is the load percentage of the wire? 

130A/50A X 100 = 260% 

Many code rules will require you to use percentages to determine wire sizes, conduit fill, circuit loading, among other things.  

Multiplier

If you need to change a number by multiplying it by a percentage, the percentage is called the multiplier. First you must convert the percentage into a decimal, and then multiply the number by the converted decimal value. 

For example: 

An over current protection device, such as a fuse or a circuit breaker, must be sized no less than 125% of the continuous load. 

For a load that is 80A, the over current protection device can not be sized less than 100A. 

To get this answer, we convert 125% to 1.25 and multiply 80 x 1.25 = 100A  

The maximum continuous load on a circuit breaker is limited to 80% of the device rating. 

For a device rated at 50A, what would be the maximum continuous load permitted? 40A. 

Why? 

80% = 0.80 

Multiply 0.80 x 50A = 40A 

Manipulating Formulas 

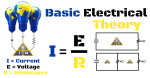
Being able to move variables around in a formula will allow you to take basic formulas and tailor them to your needs. Let’s look at the most basic electrical formula: 

V=IR 

Fundamentally, what the “=” sign means is that whatever is on the left of the sign is equivalent to whatever is on the right. So, if you change one side, you must do the same thing to the other side. So, the formula V+1=IR+1 is valid, but not very useful. (Note: when use variables, letters written next to each other means that they are multiplied together, as writing in “x” can get confusing. * also means multiply.) 

A more productive method is to eliminate parts of the formula. The base of this is that any number, when divided by itself, equals 1. (Try it on your calculator, I’ll wait). 

So, looking back at our formula: 

V=IR 

We can divide both sides by R, which gives us: 

V/R=IR/R 

Next, we know that R/R equals 1, no matter what number R actually is, so: 

V/R=Ix1 

And, multiplying anything by 1 does nothing, so we can drop the one, leaving us with: 

V/R=I, or I=V/R 

We can also combine formulas, as long as all the quantities we are talking about are the same. Let’s look at the power formula: 

P=I²R 

We can plug in I=V/R right in, giving us: 

P=(V/R)²R, or P=(V/R)*(V/R)*R 

We can eliminate one of the R/R sets from the second bracket, which leaves us with: 

P=(V/R)*V, or P=V²/R 

We can now figure out power with just voltage and resistance. 

More Electrician Math

These are just a few examples of the types of calculations you will encounter in electrician math. As the course progresses, the calculations will get more complicated. When you begin analyzing AC and three-phase circuits, you will need to know how to use trigonometric functions like sine, cosine, and tangent.

These functions can give you power factor angles, vector components, and help you fill in power triangles. Build a strong foundation and your life as an electrician will be much easier. Keep in mind that a strong foundation begins with a solid understanding of electrician math.